Optical Mineralogy
Refractive Index(
) of a substance = velocity of light in air / velocity of
light through the substance
= Vair / Vsubst.
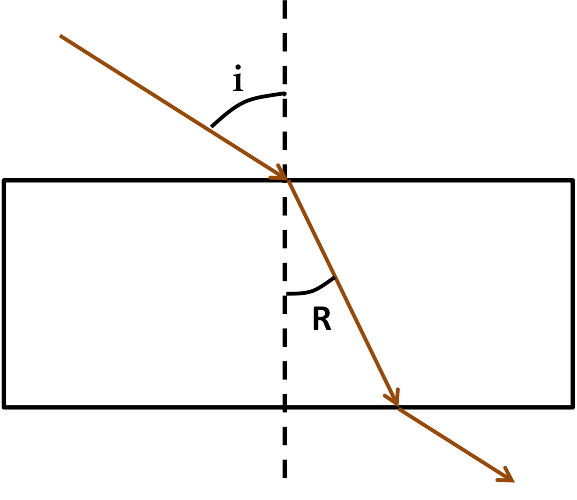
Angle of Refraction (R)
i = angle of incidence
R = angle of refraction
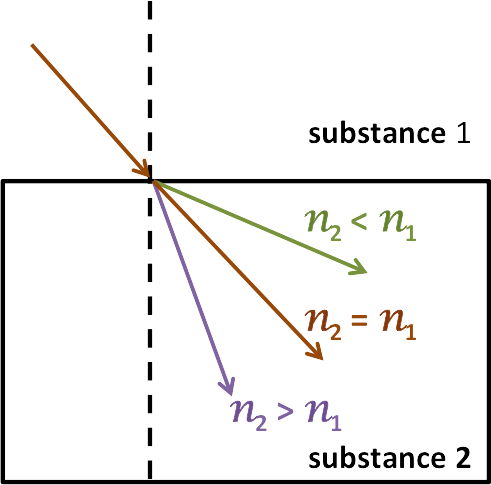
- when substances are more dense, light travels at a low velocity
& light ray bends toward the perpendicular
- when substances are less dense, light travels at a higher velocity than in denser materials
& light ray bends away from the perpendicular
Snell's Law: for calculation of refractive index (h)
from angles of incidence and refraction
Isotropic substances:
- have one refractive index because
light travels through them with the same velocity in all directions.
- Include: Isometric crystals & Amorphous materials
These substances have the same arrangement along all 3 axes => same velocity
Anisotropic substances:
- have 2 or 3 refractive indices because
light DOES NOT travel through them with the same velocity in all directions.
- Include: Hexagonal (hex/hex & hex/rhomb), Tetragonal, Orthorhombic, Monoclinic & Triclinic crystals
These substances have different arrangement along their 3 axes => different velocities
- There are 2 subcategories for anisotropic substances:
- Uniaxial: have one "optic axis" or direction along which light does
not split into two rays - all light travels at the same velocity. In
all other directions through the crystal, light splits into 2 rays with
mutually perpendicular vibration directions and different velocities.
- Includes: Hexagonal (hex/hex & hex/rhomb), Tetragonal Crystals
- Biaxial: have 2 optic axes or directions along which light does
not split into two rays - all light travels at the same velocity. In
all other directions through the crystal, light splits into 2 rays with
mutually perpendicular vibration directions and different velocities.
- Includes: Orthorhombic, Monoclinic & Triclinic Crystals
Uniaxial Ray Velocity Diagrams
Diameters reflect the VELOCITY that a light ray would have along a particular
propagation direction (direction light ray travels relative to the crystal axes).
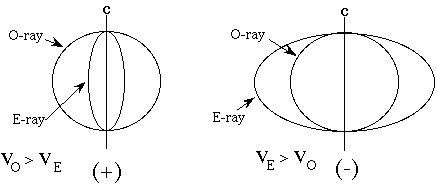Uniaxial Indicatrices
Diameters reflect the REFRACTIVE INDEX that a light ray would have along a particular
propagation direction.
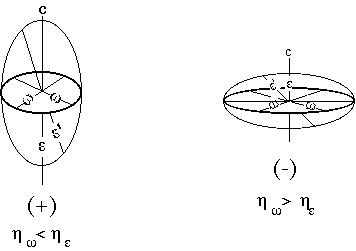
For any propagation direction (orientation of light ray passing through the crystal) the
related refractive indicies are the diameters of the cross-section which is perpendicular to the
propagation direction. In unixial crystals, one of these diameters will always be omega,
the other will be epsilon or epsilon prime.
|
http://classes.colgate.edu/rapril/geol201/
Questions to:
rapril@mail.colgate.edu
Copyright 1997 © Colgate University.
|
|
